Top How To Draw Lissajous Figures of all time Learn more here

Lissajous figures motion wave particle definition oscillatory describes sine angles right

Table of Contents

If you’re interested in creating mesmerizing geometric patterns, then learning how to draw Lissajous figures is a must-know skill. These figures are named after French physicist Jules Antoine Lissajous and are characterized by their intricate curves, which are created by the intersection of two harmonic motions. In this article, we’ll discuss how to draw Lissajous figures and provide some helpful tips along the way. So, let’s get started!

Many people struggle with creating Lissajous figures because they don’t fully understand the underlying mathematical principles. Additionally, trying to draw these figures manually can be a time-consuming and frustrating process. However, with the help of modern technology and software, it’s easier than ever to create these stunning patterns without any prior math or drawing experience.

The basic process for drawing a Lissajous figure involves plotting two harmonic motions, one for the x-axis and one for the y-axis, and then graphing the resulting curve. The amplitude and frequency of each motion will determine the shape of the resulting figure. You can adjust these parameters to create different patterns and curves.

In summary, to draw Lissajous figures, you will need to plot two harmonic motions, one for the x-axis and one for the y-axis, and then graph the resulting curve. Adjusting the amplitude and frequency of each motion will allow you to create different patterns and curves.

Creating Lissajous Figures with Desmos

Personally, I find working with Desmos, a free online graphing calculator, to be the easiest and most reliable way to create Lissajous figures. By following these steps, you can create your own Lissajous figures in no time:

1. Open Desmos.com and click on the graphing calculator option.

2. In the expression bar, input the following formula for the x-axis motion: cos(ax + b)

3. In the expression bar, input the following formula for the y-axis motion: sin(cx + d)

4. Adjust the parameters (a, b, c, and d) to create your desired figure.

Tips for Creating Lissajous Figures

Here are some tips to keep in mind while creating Lissajous figures:

• Experiment with different amplitude and frequency values to create unique and interesting patterns.
• Use different colors and line thicknesses to make your figures more visually appealing.
• Pay attention to the phase shift between the x and y motions, as this will also affect the resulting figure.

Exploring Advanced Techniques

If you’re interested in taking your Lissajous figure creations to the next level, you can explore some more advanced techniques, such as:

• Using multiple sets of harmonic motions to create more complex figures.
• Animating your figures to create mesmerizing visual effects.
• Incorporating Lissajous figures into your art or design projects.

Conclusion

Now that you know how to draw Lissajous figures, it’s time to start experimenting and creating your own unique patterns. With a little bit of practice and creativity, you can use these mesmerizing curves to enhance your art, design, and mathematical skills.

Question and Answer

Q: Can Lissajous figures be used in scientific research?

A: Yes, Lissajous figures have been used in various scientific fields, such as physics, astronomy, and engineering, to study the behavior of oscillating systems.

Q: How can I make my Lissajous figures more complex?

A: You can use multiple sets of harmonic motions and adjust the phase shift between them to create more complex patterns.

Q: Are there any other software options for creating Lissajous figures?

A: Yes, there are many software options available for creating Lissajous figures, such as GeoGebra, Mathematica, and MATLAB.

Q: What is the history behind Lissajous figures?

A: Lissajous figures are named after French physicist Jules Antoine Lissajous, who studied the behavior of pendulum systems in the 19th century.

Gallery

Curvas De Lissajous

_{^{Photo Credit by: bing.com /}}

Ej 1: Encuentre Las Ecuaciones Paramétricas Para Una Curva De Lissajous

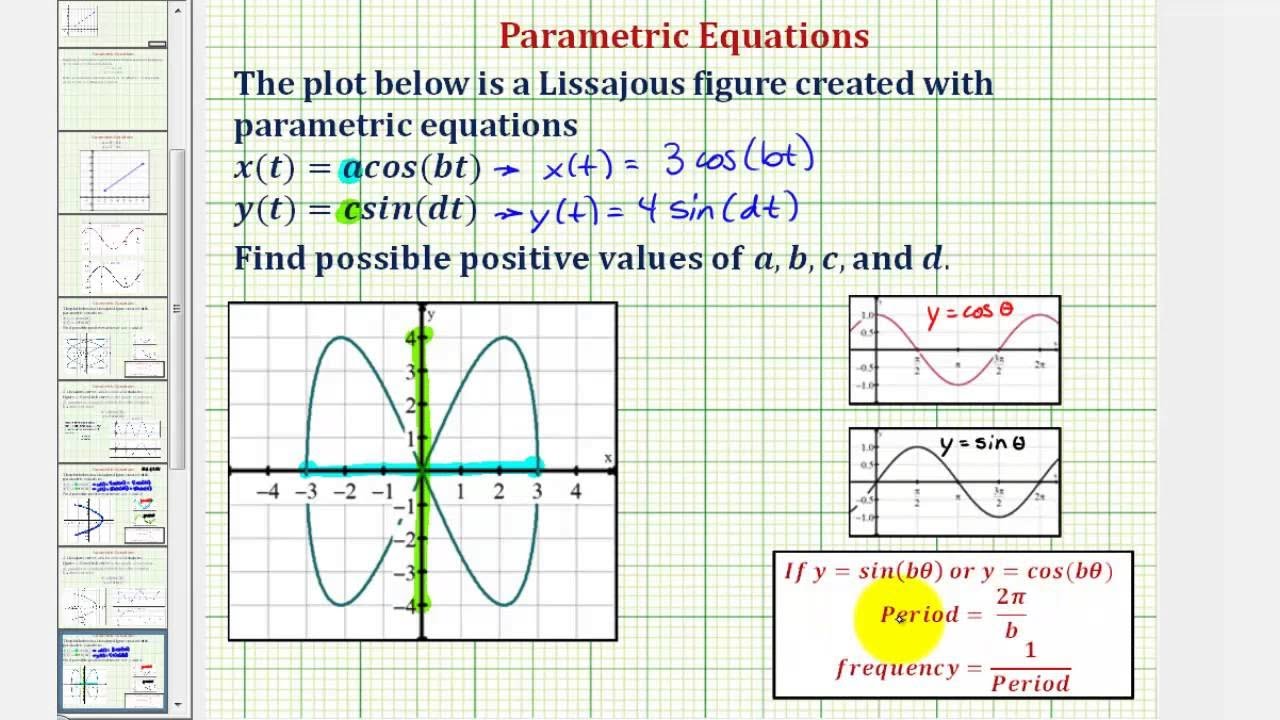
_{^{Photo Credit by: bing.com /}}

Lissajous Figures Or Lissajous Patterns - Electronics Club

_{^{Photo Credit by: bing.com / lissajous}}

Why Lissajous Figures?

_{^{Photo Credit by: bing.com / lissajous figures why}}

LISSAJOUS FIGURES

_{^{Photo Credit by: bing.com / lissajous figures motion wave particle definition oscillatory describes sine angles right}}